Unlocking the Secrets of Renormalization: A Geometrical Formulation That Will Blow Your Mind!

If you are fascinated by the underlying principles of physics and mathematics, then you must have come across the concept of Renormalization Group (RG) method. Considered a powerful tool in theoretical physics, RG allows us to understand the behavior of physical systems at different scales. In this article, we will delve into the geometrical formulation of the Renormalization Group method as an asymptotic and explore its various applications.

Understanding Renormalization Group Method

The Renormalization Group method is a mathematical technique used to study systems with multiple scales. Whether it's understanding the behavior of particles in quantum field theory or analyzing phase transitions in condensed matter physics, RG provides invaluable insights into how physical systems evolve under different energy scales.

In the simplest terms, the RG method involves coarse-graining a system, which means examining it at a particular resolution or scale. By systematically analyzing the effects of integrating or removing high-energy degrees of freedom in the system, we gain a better understanding of its behavior at larger scales.

Geometrical Formulation of Renormalization-Group Method as an Asymptotic Analysis: With Applications to Derivation of Causal Fluid Dynamics (Fundamental Theories of Physics Book 206)

by Temitope James(Kindle Edition)

5 out of 5

Language	:	English
File size	:	146735 KB
Text-to-Speech	:	Enabled
Screen Reader	:	Supported
Enhanced typesetting	:	Enabled
Word Wise	:	Enabled
Print length	:	810 pages

FREE

The Geometrical Formulation

A key insight that has revolutionized the understanding of RG is the geometrical formulation. Using the language of differential geometry, we can represent the RG transformation as a flow through a high-dimensional space. This formulation allows us to visualize the evolution of a system's parameters as we change the energy scale, providing us with a geometric interpretation of the RG method.

Imagine a multiverse of all possible theories and each theory represented as a unique point in a high-dimensional space. As we change the energy scale, the RG flow takes us on a journey through this theoretical landscape. Points that are close together in this space represent similar theories, while distant points represent radically different physical systems. This geometrical picture helps us understand the universality of certain phenomena and the emergence of critical behavior in systems.

Applications of the Geometrical Formulation

The geometrical formulation of the RG method has found numerous applications in various branches of physics, including quantum field theory, statistical mechanics, and condensed matter physics. Let's explore some of the key areas where this formulation has proven to be invaluable.

Quantum Field Theory

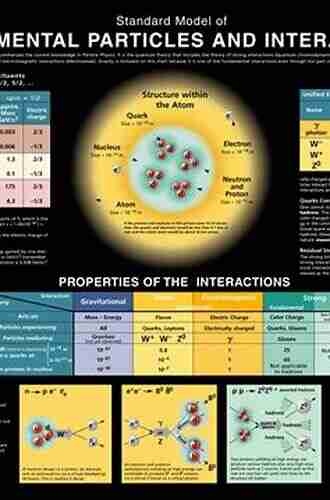
In quantum field theory, the RG offers a framework to understand the behavior of particles and interactions at different energy scales. By analyzing the flow of couplings and parameters in the theory's Lagrangian, we can determine its ultraviolet and infrared fixed points. The geometrical formulation allows us to study the renormalization group flow as trajectories on the energy scale manifold, enabling us to identify the universality classes of different theories.

Statistical Mechanics

When studying phase transitions in statistical mechanics, the RG method provides a powerful tool to understand how collective behavior emerges from the interactions of individual particles. By mapping the theory onto a lattice and applying the RG transformation, we can study the flow of the effective Hamiltonian and identify the critical exponents characterizing the phase transition. The geometrical formulation helps us visualize the RG flow and understand the critical phenomena in a geometric context.

Condensed Matter Physics

In condensed matter physics, the RG method aids in understanding the behavior of materials at different length scales and under different external conditions. From understanding the scaling properties of the electronic structure to analyzing the emergence of topological phases, the geometrical formulation allows us to explore the phase diagram of complex materials systematically.

The geometrical formulation of the Renormalization Group method as an asymptotic has unlocked new vistas in theoretical physics. By providing a visual and intuitive representation of the RG flow, it has enhanced our understanding of the behavior of physical systems at different scales. From quantum field theory to statistical mechanics and condensed matter physics, the RG method has found widespread applications across numerous fields. So, the next time you encounter a system with multiple scales, remember the power of the geometrical formulation that lies at the heart of the Renormalization Group method!

Geometrical Formulation of Renormalization-Group Method as an Asymptotic Analysis: With Applications to Derivation of Causal Fluid Dynamics (Fundamental Theories of Physics Book 206)

by Temitope James(Kindle Edition)

5 out of 5

Language	:	English
File size	:	146735 KB
Text-to-Speech	:	Enabled
Screen Reader	:	Supported
Enhanced typesetting	:	Enabled
Word Wise	:	Enabled
Print length	:	810 pages

FREE

This book presents a comprehensive account of the  renormalization-group (RG) method and its extension, the doublet scheme, in a geometrical point of view.

It extract long timescale macroscopic/mesoscopic dynamics from microscopic equations in an intuitively understandable way rather than in a mathematically rigorous manner and introduces readers to a mathematically elementary, but useful and widely applicable technique for analyzing asymptotic solutions in mathematical models of nature.

The book begins with the basic notion of the RG theory, including its connection with the separation of scales. Then it formulates the RG method as a construction method of envelopes of the naive perturbative solutions containing secular terms, and then demonstrates the formulation in various types of evolution equations. Lastly, it describes successful physical examples, such as stochastic and transport phenomena including second-order relativistic as well as nonrelativistic fluid dynamics with causality and  transport phenomena in cold atoms, with extensive numerical expositions of transport coefficients and relaxation times.

Requiring only an undergraduate-level understanding of physics and mathematics, the book clearly describes the notions and mathematical techniques with a wealth of examples. It is a unique and can be enlightening resource for readers who feel mystified by renormalization theory in quantum field theory.